On this day in 1558, Mary Tudor, Queen of England, consort of Philip II of Spain, died at St. James Palace, and was succeeded by her half-sister, Elizabeth. In 1869, the first ships officially passed through the Suez Canal in Egypt, creating a more efficient trade route for the countries along the Indian Ocean and those along the Mediterranean.
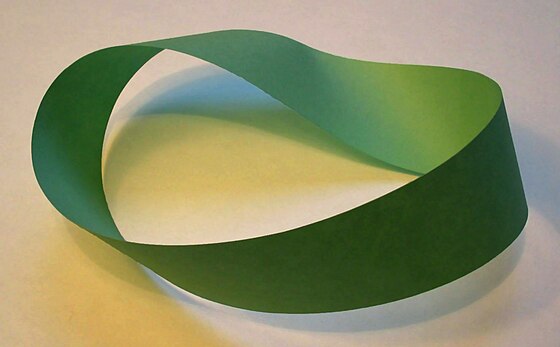
This day is also the birthday of August Ferdinand Möbius, in 1790 in Saxony, Germany. A descendant of Martin Luther, homeschooled into his teens, he started college at thirteen, graduating six years later and then going on to study astronomy and mathematics from Carl Friedrich Gauss. While he did significant work in astronomy, he is best known for his discovery of the Möbius strip, described as “a non-orientable two-dimensional surface with only one side when embedded in three-dimensional Euclidean space.”
One of the field trips we took in fourth grade was to the California Museum of Science and Industry in Los Angeles (long since demolished to make way for the California Science Center). In the Hall of Mathematics was a model of this figure, with an arrow on a track. Pushing a button sent the arrow along the strip, proving that it had only one side. We came back from the field trip and were given strips of paper and tape so that we could make our own strips and draw on them to prove to ourselves that a mathematical figure, which looked two-sided and its surfaces disconnected, was in fact a single-sided figure.
We are often confronted with the realization that many situations involve two sides of the same issue. Möbius’ strip raises the possibility that perhaps, sometimes, they are actually the same side, completely connected and integrated, and only our perception divides them, as the issues of religion divided Mary and Elizabeth, who now share the same tomb in Westminster Abbey, and the desert sands divided the Mediterranean Sea and the Indian Ocean, whose waters now mingle in the Suez Canal locks.